With the start of the second semester, the focus in math has shifted from working with whole numbers to numbers that are less than one whole; also known as fractions.
The main learning objective currently is the Common Core State Standard 4.NF.2:
4.NF.2. Compare two fractions with different numerators and
different denominators, e.g., by creating common denominators or numerators, or
by comparing to a benchmark fraction such as 1/2. Recognize that comparisons are valid only
when the two fractions refer to the same whole. Record the results of
comparisons with symbols less than, greater than or equal to, and justify the conclusions, e.g.,
by using a visual fraction model.
To make the concept a little easier to understand, students are using fraction models.
These models allow students to better visualize the fractions being used and to think about their size relative to other fractions and to one whole. This is especially helpful when students are asked to compare fractions or find equivalent fractions.
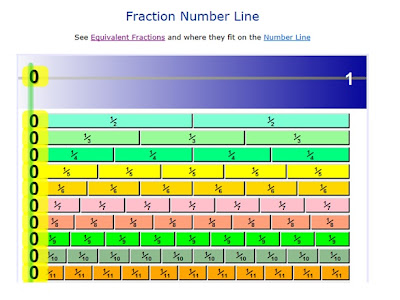
This particular web based model is really nice because it allows students to move around on the number line. It automatically shows when there are other fractions that are equivalent to the one a students lands on.
Here is a picture of it. Click on the picture to try it out.
You can easily see at the top of the image that the student has chosen the fraction 2/3. Then if you follow the green line down, all of the equivalent fractions to 2/3 on the chart are indicated.
Comparing Fractions
It is easy to compare fractions with the same (or like) denominators. Let's use this example:
When comparing fractions with like denominators, the focus moves to the numerator. In the example above, both fractions are broken into 7 parts. The fraction with 2 parts shaded in is clearly smaller than the one with 5 parts shaded in. Therefore two-sevenths is less than five sevenths.
When comparing fractions with unlike denominators, one must really think about and try to picture the fractions. We don't always have pictures in front of us. It is okay to represent the fractions with a picture model, but it is also importnat to have mathematical strategies other than pictoral.
One strategy students have been taught is to compare fractions to the "benchmark" of 1/2. A benchmark fraction is an easily identifiable fraction students can use to help them identify or mark other fractions.
By representing the fractions with a model, students can easily draw both fractions and clearly see that the two fractions are equal to each other in size. When different fractions are the same size, we call them equivalent fractions. We will be discussing these more at a later time.
In addition to representing fractions with a model, students should have a mathematical strategy they can use to solve problems. In the above example, one would first look at the denominator, or bottom number. Then, one would think about the numerator, or top number.
The question one should ask is "Is the numerator more than half, less than half or equal to half of the denominator?" In this case, 3 is half of 6, so that tells us this fraction is equal to half. Let's look at another example...
Students can use pictures models or use mathematical strategies to compare fractions to one half. If a student is comfortable with these strategies, she or he can now use them to compare fractions with unlike denominators.
Here is a video showing how to use these strategies to compare fractions with unlike denominators.
Please comment! How is Fractions going for you? What have you learned? What are you still trying to learn? What strategy works best for you when comparing fractions?